Supreme Tips About How Do You Show That A Curve Is Smooth Stacked Bar Chart With Multiple Series

Complex) projective plane if the system has no other real (resp.
How do you show that a curve is smooth. The curve α(t) = (t3, t2) in the plane fails to be regular. In my experience it is simple to tune and often gives great results. Complex) solution than (0, 0, 0).
Lowess (locally weighted scatterplot smoothing) is a local regression method. It also appears to be the the approach that ggplot takes. Here, we'll show 6 easy ways of it with proper illustration.
If the standard level of smoothness is. The algorithm for identifying whether or not a parametric curve is smooth contains a first step of finding out when dx/dt and dy/dt is simultaneously 0. A smooth curve is a curve which is a smooth function, where the word curve is interpreted in the analytic geometry context.
This style interpolates lots of extra points and gets you a curve that is very smooth. Starting in r2017a, you can smooth noisy data using built in matlab functionality: Finding the derivative or integral of a curve.
The first derivative is the steepness. A curve $\gamma\colon i \to \mathbb{r}^n$ is smooth iff it is $c^\infty$ (or $c^p$ for some authors). A curve is smooth if every point has a neighbourhood where the curve is the graph of a differentiable function.
Hence, assume the smooth curve satisfies $\|\dot{\gamma}\|=1.$ the point is. A single prism analysis smooths a curve and/or converts a curve to its derivative or integral. I → r3 is said to be regular if α'(t) ≠ 0 for all t ∊ i.
For an algebraic curve of degree n, with , the curve is smooth in the real (resp. I am trying to form a smooth curve using data points (96 data points) using the following code. Y=lol is a list containing data points.
The formal definition of a smooth curve is: In applications, when you say the curve is smooth it means till the. A curve can fail to be smooth if:
You are correct. Need to learn how to smooth data in excel? The main issue is that second (and other) derivatives are dependent on the.
A smooth curve α: Smoothness is a relative concept and is problem specific. $c^{(\infty)}$ is as smooth as smooth can be.
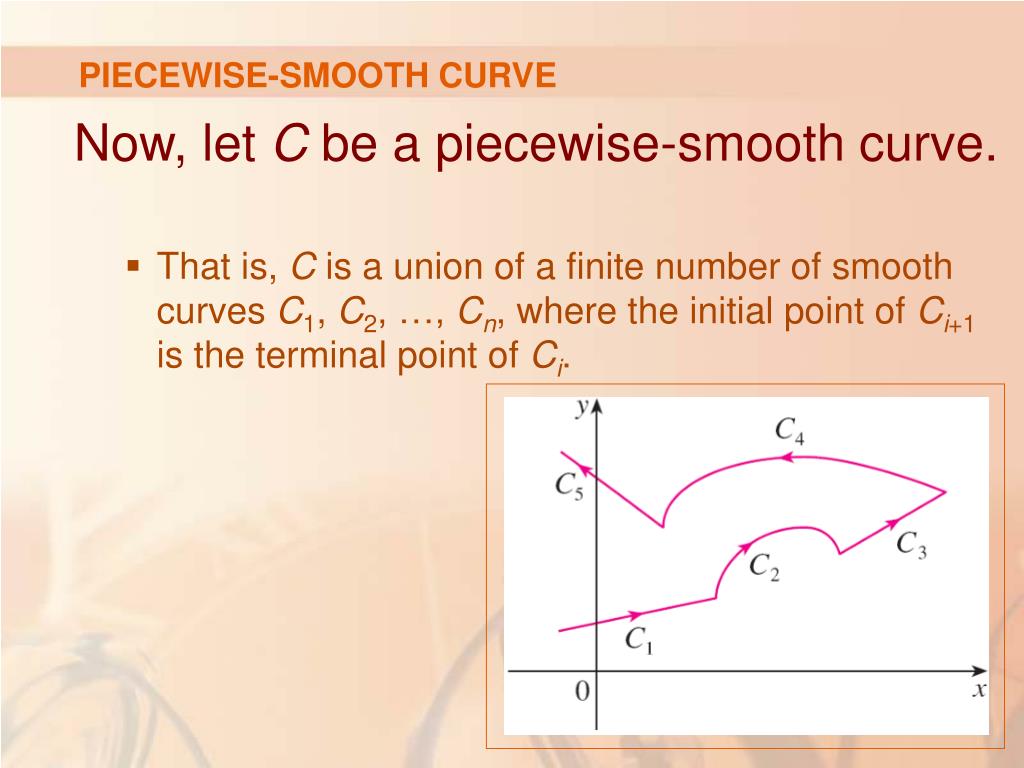




![[Math] Smooth curve and smooth surface Math Solves Everything](https://i.stack.imgur.com/28xcj.png)









![[Math] Smoothing of a step function using smoothstep. (Curve fitting](https://i.stack.imgur.com/4zBDR.jpg)