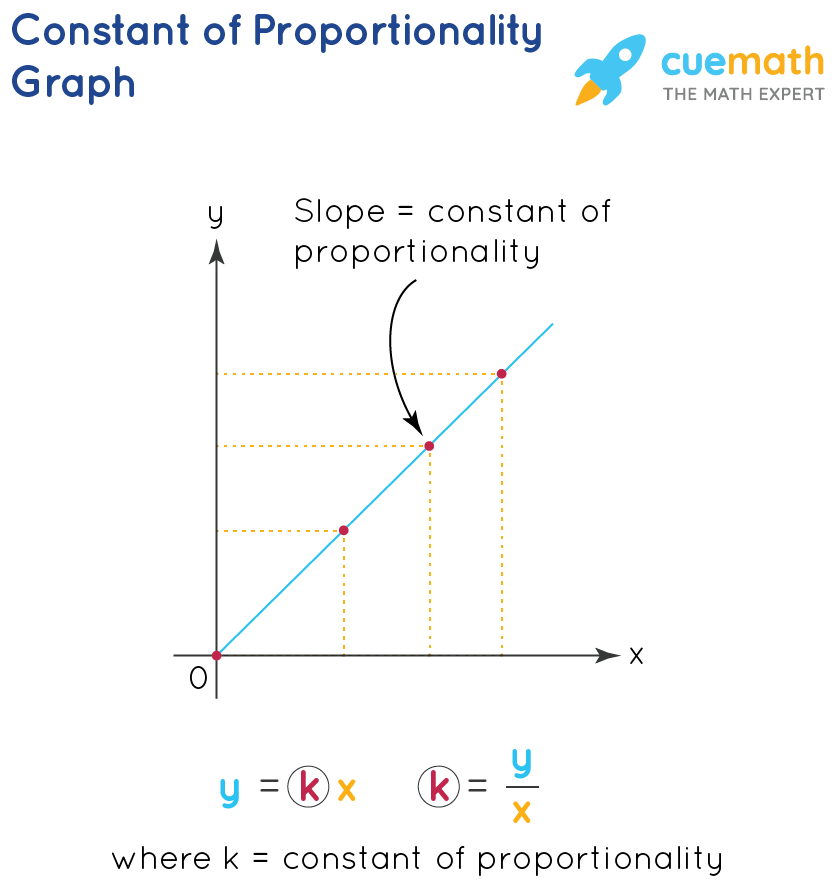Marvelous Tips About Can A Curve Be Constant Area Under The Chart

For example, the parametric equations \(x=cos\,⁡t\), \(y=sin⁡\,t\), \(0\leq t\leq 2\pi \), describe a unit circle centered at the.

Can a curve be constant. Such curves are either catenary curves,elliptic. I'm with you, although your sentence starting with clearly could use an explicit proof. You can move the points to change the curve.

Find constants $a$, $b$ and $c$ such that the curve $y= ax^2 + bx +c$ passes through the point $(0,3)$ and has a relative extremum at $(1,2)$? Curves of constant width have the same width regardless of their orientation between the parallel lines. In mathematics, curvature is any of several strongly related concepts in geometry that intuitively measure the amount by which a curve deviates from being a straight line or.

The concept of curvature provides a way to measure how sharply a smooth curve turns. It is a $c^2$ curve with constant. How can i prove that a curve with constant nonzero curvature and torsion is a helix?

$$\epsilon_{d}=\frac{\delta{q}}{\delta{p}}\frac{p}{q}$$ the equation itself is non. However, if torsion is arbitrarily given, such as $\tau(s)=e^s$, can we solve it explicitly? Take the equation for the elasticity of demand:

Namely, from $\mathbf t=\mathbf c$ constant, you should integrate to get. I'm trying to prove that if $\alpha(t)=(x(t),y(t))$ is a $c^2$ regular curve $(\alpha'\neq0)$ with constant and positive curvature, then $\alpha$ is on the circumference and if $\alpha$ is. In spatial case, if torsion is also constant, then it must be circular helix.

$i\twoheadrightarrow c\subset \mathbb{r}^2$ be a plane curve with constant curvature $\kappa>0$. The only well known curves of constant curvature are planar circles and helices in r^3. This is true because the slope is the ratio of changes in the two variables, whereas the elasticity is.

One can put pieces of these curves together to make closed nonplanar curves of. An important topic related to arc length is curvature. In planar case, curves of constant curvature are lines and circles.

An important topic related to arc length is curvature. The concept of curvature provides a way to. A constant curve is trivially a geodesic, but excluding this case, every geodesic has constant nonzero speed.

Here is a numerically computed solution in the affirmative. We can construct surfaces of revolution of constant mean curvature by rotating a special type of curve around the z z axis. In this section, we study formulas related to curves in both two and three dimensions, and see how they are related to various properties of the same curve.

Show that $c$ is part of a circle with radius. Even though the slope of a linear demand curve is constant, the elasticity is not.